Written by: Karthik Prasad

Seven Problems. 1 million dollars for each one solved. The Yang-Mills Mass Gap problem is one of the fabled Millenium Problems and is of great interest in mathematical physics. Its statement is…incomprehensible for most, filled with abstract terms: prove that for any compact, simple gauge group G, a non-trivial quantum Yang–Mills theory exists on R4 and has a mass gap Δ > 0. Existence includes establishing axiomatic properties at least as strong as those cited in Streater & Wightman (1964), Osterwalder & Schrader (1973), and Osterwalder & Schrader (1975). In this article, we discuss what all of those terms in the problem mean, what the problem actually is, and why it is important.

Quantum Field Theory

To understand the Yang-Mills problem, we must first understand what it is asking about. Quantum Field Theory (or QFT) is our most modern descriptor of quantum phenomena and accurately describes most predictions of quantum mechanics and special relativity. It operates under the principle that there are certain “fields” for every type of particle, and individual particles are excitations of this field. Then, techniques from differential geometry and other areas of mathematics and physics can be applied to determine how these fields interact. Fundamentally, the Yang-Mills Problem asks questions about quantum field theories—specifically, whether a four-dimensional QFT with a mass gap (which we will discuss later) exists. 

Figure 1

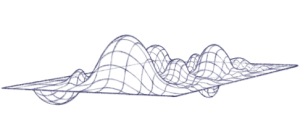
Depiction of a Field with Various Excitations

Source: [Quanta Magazine]

The Problem with QFT

Quantum Field Theory, however, has an issue. When certain physical quantities are computed, they produce infinities, which is unreasonable. In the physical world, a quantity of infinity does not make sense – there is a finite amount of mass, speed, size, etc. for objects. A box of toys cannot have infinite mass or infinite velocity, and therefore, the infinities of QFT do not match up with reality.

The process of renormalization, where these infinities are “subtracted,” is a solution—however, this process is mathematically unrigorous. A rigorous QFT is a quantum field theory that does not have these unrigorous mathematical procedures and results within it: in other words, everything that the QFT does is mathematically “proper”. 

Mathematicians for years have been attempting to produce a rigorous quantum field theory, and in 1964, Arthur Wightmann produced a set of axioms that any rigorous QFT must satisfy, called the Wightmann axioms. The Yang-Mills problem attempts to produce an axiomatic, rigorous QFT and therefore imposes the requirement that the solution must fulfill the Wightmann Axioms.

The Mass Gap

In a quantum field theory, a mass gap Δ is essentially the mass (where mass includes the fundamental energy of the particle via mass-energy equivalence) of the lightest particle in the QFT. This is in general important because with a mass gap Δ = 0, there must be at least one massless particle in the QFT. But, physicists have not discovered any massless particles in the real world, and therefore any QFT modeling our predictions must have a mass gap greater than 0. 

Why is Yang-Mills Important?

For the Yang-Mills problem, we specifically consider Yang-Mills theory, a special type of QFT. Yang-Mills theories are essentially QFTs that are based on special algebraic structures with certain properties. 

Notably, a fourth-dimensional Yang-Mills theory is the simplest nontrivial—where nontrivial means not obvious or overly basic —QFT that can be constructed in four dimensions. This is useful because, as the Yang-Mills theory is nontrivial, there are many interesting implications and studies that can be done with it that cannot be done with trivial theories. 

But, because it is the simplest 4-dimensional QFT, it is easier to study than other examples. In particular, it has been unrigorously shown via theoretical physics that Yang-Mills theory exhibits a property called quantum confinement, which essentially places restrictions on particles. These restrictions apply to gluons, which are manifestations of the strong nuclear force. These restrictions force gluons (which are massless by themselves) into composite structures called glueballs that have a distinct mass > 0 associated with them. These glueballs turn out to be the lightest particles in Yang-Mills and therefore imply a mass gap Δ > 0.

Figure 2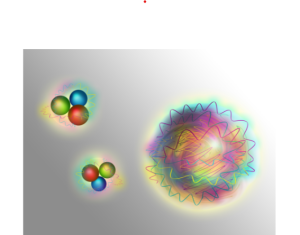

Depiction of individual gluons and a composition of gluons, a glueball

Source: [Phys.org]

Then, essentially, providing a solution to the Yang-Mills Problem shows a mathematically rigorous (Wightmann axioms) approach to the fourth-dimensional Yang-Mills theory that matches physicists’ measurement of only massful particles in the real world (mass gap > 0). Such a solution would allow mathematicians to make much more accurate and interesting observations about Yang-Mills theory and extend the techniques used to other quantum field theories and problems in differential geometry, making it very important for their understanding of QFT and mathematics.

Conclusion

In this article, the ideas behind the Yang-Mills Mass Gap problem are discussed. Quantum field theories—based around the concept of particles being excitations in fields—are described, as are the attempts to axiomatize QFT through the Wightmann axioms. The fourth-dimensional Yang-Mills theory and its unrigorous implications of a mass gap are explained, allowing one to understand the statement of the Yang-Mills Millenium Problem. Finally, the importance of mathematicians solving this problem and discovering new techniques and results from the 4-dimensional Yang-Mills theory is discussed.

References and Sources

1:  Strogaz, S. (2022, August 10). What Is Quantum Field Theory and Why Is It Incomplete? | Quanta Magazine. Quanta Magazine. https://www.quantamagazine.org/what-is-quantum-field-theory-and-why-is-it-incomplete-20220810/

2.  Technology, V. U. of. (2015, October 13). A particle purely made of nuclear force. Phys.org. https://phys.org/news/2015-10-particle-purely-nuclear.html
3.  Jaffe, A., & Witten, E. (n.d.). QUANTUM YANG-MILLS THEORY. Retrieved December 1, 2023, from https://www.claymath.org/wp-content/uploads/2022/06/yangmills.pdf